Investment Incentives And Market Power: An Experimental Analysis
| This document is available in two formats: this web page (for browsing content and, PDF (comparable to original document formatting). To view the PDF you will need Acrobat Reader, which may be downloaded from the Adobe site. |
|
ECONOMIC ANALYSIS GROUP DISCUSSION PAPER
EAG Discussion Papers are the primary vehicle used to disseminate research from economists in the Economic Analysis Group (EAG) of the Antitrust Division. These papers are intended to inform interested individuals and institutions of EAG's research program and to stimulate comment and criticism on economic issues related to antitrust policy and regulation. The analysis and conclusions expressed herein are solely those of the authors and do not represent the views of the United States Department of Justice. Information on the EAG research program and discussion paper series may be obtained from Russell Pittman, Director of Economic Research, Economic Analysis Group, Antitrust Division, U.S. Department of Justice, BICN 10-000, Washington, DC 20530, or by e-mail at russell.pittman@usdoj.gov. Comments on specific papers may be addressed directly to the authors at the same mailing address or at their e-mail address. Recent EAG Discussion Paper titles are listed at the end of the paper. To obtain a complete list of titles or to request single copies of individual papers, please write to Janet Ficco at the above mailing address or at janet.ficco@usdoj.gov or call (202) 307-3779. Beginning with papers issued in 1999, copies of individual papers are also available from the Social Science Research Network at www.ssrn.com. Abstract We examine investment incentives and market power in an experimental market. We characterize market power as the strategic interdependence of subjects' investment decisions and output decisions. The market is designed so that investment and output decisions can be jointly characterized as strategies within a game. A Nash-Cournot equilibrium of the game provides a way of characterizing how investment incentives and market power interact. Subjects could invest in two different production technologies and could produce output to serve as many as two different demand conditions. The technologies were analogous to "baseload" capacity and "peaking" capacity in wholesale electricity markets. The Nash-Cournot benchmark constituted a good indicator of subjects' output decisions in that output cycled around the Cournot benchmark. Thus, on average, consumers extracted the surplus available to them in the equilibrium. While we do not observe Edgeworth Cycles in prices or outputs, we do see them in the producer surplus series. Producers dissipated some of the surplus they could have extracted in the equilibrium by overinvesting in peaking capacity and underinvesting in baseload capacity. Inefficient investment diminished total system efficiency, but producers' investments in total production capacity tracked the Nash-Cournot benchmark. In contrast, monopoly explanations such as collusion do not characterize the data. keywords: capacity investment, Cournot, supply function equilibrium, Edgeworth Cycles, market power, electricity markets, investment incentives JEL codes: C92, D43, C72 1. Introduction In 1993 Charles Plott observed that "Designer markets are becoming a reality." (Plott 1994, pg. 3) Since then, market design has become big business, so much so that the topic has migrated, under various guises, from the theorist's notepad, to the policymakers' forum, to the front page of any newspaper. Casual consumers of news may, for example, know something about the market-based "cap-and-trade" mechanisms proponents of the Kyoto Protocol on global warming have engineered. They may also know something about the auction mechanisms government agencies have used for allocating rights to radio spectra or about schemes municipalities have been implementing to mitigate traffic congestion. Yet others may have experience with the efforts of regulators to create wholesale electricity markets. All of these applications pollution abatement, spectrum auctions, network congestion, and wholesale electricity constitute just a few of the allocation problems within the designer's purview. Part of the appeal of actively designing markets is that it may allow parties to (1) achieve superior allocative efficiency and to (2) achieve it in environments that are hostile to the spontaneous emergence of markets. Banks, Ledyard and Porter (1989) discuss environments in which exchange mediated in institutions that look a lot like traditional markets may be difficult to operationalize. They observe that even when one can organize traditional market institutions, the allocative efficiency of markets may not dominate that of centralized, administrative means of organizing exchange. But that just raises the ante for designers. The authors' principal point is that scope may yet remain for designing (possibly non-traditional) market rules to improve the performance of decentralized exchange mechanisms. In designing rules governing exchange in wholesale electricity markets, policymakers have endeavored to design rules that would frustrate the exercise of market power (Wolak and Patrick 2001). Even so, one of the principal motivations for "restructuring" wholesale electricity markets was explicitly dynamic: to give energy firms incentives to invest over time in new, cleaner, more efficient generation and transmission capacity, all in an effort to move electrons and get the lights on at lower cost (Roques, Newbery and Nuttall 2005; Blumstein, Friedman and Green 2002). More efficient producers would be better situated to capture gains from trade in day-to-day exchange mediated in restructured markets; those gains would create opportunities and incentives to invest. Less efficient capacity would prove unprofitable to maintain; firms would substitute more efficient capacity for less efficient capacity. In the process both producers and consumers would gain from increased efficiency. Electricity market restructuring did induce investment in new generation capacity, but the day-to-day experiences in restructured markets sometimes proved to be problematic or, at least, politically incorrect. In some applications allocative efficiency may be decomposed into static and dynamic dimensions; market rules (and changes to them) can have both static and dynamic effects. One static consideration is market power, in which the primary concern is whether the market rules enable participants to raise short-run prices by reducing output. Among other things, trade in restructured markets exhibited "price spikes," seen most notably in California in 2000-2001. These price spikes have been attributed to the ability of generators to exercise market power (Borenstein, Bushnell, and Wolak 2002; Joskow and Kahn 2002). It is not obvious that price spikes are pathological per se, because price increases grounded in true scarcity provide valuable investment signals, but they have attracted negative attention. Even if one can attribute price spikes to the exercise of market power, there may be interactions between investment behaviors and such manifestations of market power. How such interactions can inform the design of markets remains poorly understood. On one hand, the motivation to restructure markets rests on dynamic considerations (investment over time), but the seemingly pathological results experienced in restructured markets highlights static considerations (market power). The research presented in this paper shifts focus to broader theoretical questions about relationships between investment incentives and market processes and presents some evidence on these relationships in experimental markets. The paper presents a model that enables characterization of interactions between investment and market power. Do investment and market outcomes correspond to theoretical benchmarks? Do they systematically deviate from theoretical benchmarks? Do market participants tend to invest beyond theoretical benchmarks? Do they systematically underinvest? Are certain market designs more conducive to investment than others? Ultimately, can we generate discrete results about the interactions of investment incentives and market power that can inform market design? We characterize investment incentives and market power in a simple model, and we use that model to guide the design of an experiment. One great advantage of the model is that it provides a way of operationalizing the concept of market power. It allows us to characterize market power as the strategic interdependence of parties' investment decisions and output decisions. In turn, the appeal to strategic interdependence allows us to characterize competition among parties who possess market power as a game. Equilibria of the game constitute predictions of how investment behaviors and market processes interact. The model borrows two salient features of electricity markets: parties can invest in more than one production technology, and they may compete in markets featuring different demand conditions. The production technologies are analogous to "baseload" and "peaking" capacity in energy markets, and the demand conditions are analogous to "off-peak" demands and "peak" demands in those same markets. The structure of the game provides a simple way of characterizing parties' investments in more than one production technology. A striking feature of the experimental design is that it allows us to characterize those investments without having to appeal to other, real-world features of energy technologies such as "ramp rates," startup costs, and shutdown costs features that might distract analysis from the more fundamental considerations. We can characterize behaviors in the experimental markets as Cournot, and we craft a Cournot benchmark and other theoretical benchmarks to characterize how well experimental subjects invest. The principal results are that the Cournot benchmark constitutes a good indicator of subjects' output decisions and, accordingly, that the system generates Cournot-consistent consumer surplus. Subjects extract less than the Cournot-consistent producer surplus, because they tend to overinvest in peaking capacity and underinvest in baseload capacity. The data do not support monopoly explanations such as collusion for subjects' behaviors. The Cournot benchmark also constitutes a better predictor of behaviors than the benchmark corresponding to socially optimal investment and outputs. The existing experimental framework allows us to generate a number of important results, but it also constitutes an expandable platform for analysis of more complex questions. It does not yet accommodate questions about investment over time or questions about investment in new technologies, although it can be easily extended to do so. Indeed, extending the framework will be the object of immediate follow-on research. In the framework applied here, subjects participated in a sequence of games, and we can interpret each game as a stage game of a larger, repeated game. The technologies remained fixed across all of the stage games, and each stage game was static in that subjects' investment decisions did not roll over into succeeding stages. As it is, the existing framework allows us to identify important dynamic phenomena such as "sawtooth patterns" or "Edgeworth Cycles" (Maskin and Tirole 1988) in the data. The remainder of the paper proceeds in five parts. The first part reviews literature involving applications of Nash-Cournot hypotheses. The second part presents a model we used to inform the design of the experiment, the third part features the experimental design, and the fourth part presents the results of the experiment. The final part concludes. 2. Related Literature The research presented here relates to three broad lines of research: (1) empirical research on oligopolistic competition in wholesale electricity markets, (2) experimental research on oligopolistic competition in quantity-setting environments, and (3) "dynamic oligopoly" more generally. The first illuminates the advantages and limits of Cournot hypotheses in evaluating electricity markets. The second provides some basis for evaluating Cournot hypotheses themselves and extends analysis to competition that joins quantity-setting behaviors with price-setting behaviors. The third body of literature points up dynamic considerations that hypotheses about behaviors in a static environment, including Cournot hypotheses, cannot accommodate. Research on the performance of wholesale electricity markets suggest that, both as a matter of theory and practice, Nash-Cournot hypotheses constitute conservative benchmarks. The implication is powerful: Posing Nash-Cournot behaviors allows analysts to characterize worst-case scenarios. Worst-case scenarios constitute conservative forecasts of market performance. Thus, policymakers and industry analysts can use these Cournot-consistent forecasts to benchmark prospective changes in the allocation of industry production capacity. Such changes might attend discrete events like mergers, new entry, or market restructuring. Borenstein and Bushnell (1999) explicitly appealed to such reasoning when forecasting the performance California's restructured electricity market (pp. 289-291). They were interested in evaluating one of the principal features of the "restructuring": the divestiture of electricity generation capacity from vertically-integrated utilities to vertically-separated generating firms. They used measures of firms' marginal costs and imposed a demand function featuring constant elasticity. Their simulation of market performance amounted to imposing different elasticities of demand and calculating the Cournot-consistent outputs those elasticities implied. Posing Cournot behaviors allowed them to benchmark market performance that would succeed divestiture, and, presciently they suggested that the availability of "hydro[electric] power in the Pacific northwest and California plays a large role in determine the extent and severity of market power." (pg. 299) By the summer of 2000, drought had denied California much of the hydroelectric generation upon which it had depended, contributing to "price spikes" experienced in wholesale electricity markets. Borenstein and Bushnell observe that empirical research on electricity markets has appealed to different models: the "supply function equilibrium" approach (SFE) innovated by Klemperer and Meyer (1989), Cournot models, and auction models. (pp. 288-289) Klemperer and Meyer observe that Cournot constitutes a special, worst case of SFE, a fact that Borenstein and Bushnell use to substantiate their appeal to Cournot. The great advantage of Cournot, of course, it its tractability in understanding strategic interdependence of producers' output decisions. SFE is more general because it goes some way toward accounting for price-setting behavior; the concept of bidding entire "offer curves" more accurately reflects exchange in markets such as wholesale electricity markets. In contrast, Cournot relies implicitly on an unmodeled market institution that allocates output to parties on the demand side. Yet, while SFE is more general, there is a familiar tradeoff: Cournot "allows much more detailed modeling and determinacy in solutions than the supply curve bidding approach." (Borenstein and Bushnell 1999, pg. 290) Borenstein and Bushnell go on to observe that Cournot constitutes a "worst-case (static equilibrium) scenario" under SFE the suggestion being that it constitutes a conservative benchmark of performance. Other research has exploited the more general concept of SFE. Green and Newbery (1992) extended SFE to accommodate supply constraints so that they could apply SFE to electricity markets. Their approach is interesting, because supply constraints constitute an important condition in electricity markets, but their SFE model leaves open the prospect of extending the static analysis of markets to an analysis that is dynamic in that it accounts for the endogeneity of those constraints. While SFE provides a theoretical basis for the proposition that Cournot constitutes a conservative benchmark, the question of whether or not Cournot is conservative is ultimately empirical. It turns out there is some empirical evidence consistent with the proposition. Wolfram (1999) observes that outcomes in the British market were not as bad as SFE (and thus, Cournot) suggest they could have been. Specifically, prices were not as high as SFE and Cournot simulations suggest they could have been. An affirmative interpretation of her results is consistent with the Borenstein and Bushnell approach: Cournot may not exactly characterize behaviors but, as a matter of theory and practice, it constitutes a conservative bound. The proposition that Cournot constitutes a conservative benchmark for evaluating market performance is empowering. It suggests that if posing the Nash-Cournot hypothesis allows researchers to indicate that markets are performing well, then they need not agonize over the prospect of operationalizing more ambitious hypotheses. Even so, one might hope that one could appeal to other empirical research to evaluate the proposition. Wolfram herself suggested that "It is unclear to what extent one can draw implications from the experience in the British [wholesale electricity market] for electricity industry restructurings elsewhere." (pg. 822) Wolfram observed that it is not obvious why prices observed in the British were lower than benchmark models predicted and went on to suggest that idiosyncratic features of the British market rather than systematic features of behaviors in markets could account for the results. The question of whether or not Cournot poses a conservative benchmark can be tested using the experimental method. While no experimental research has explicitly taken up this question, there is abundant research on competition in quantity-setting environments. Much of this research also joins quantity-setting competition with price-setting competition. The point of departure for much of this experimental research is the hypothesis of Kreps and Scheinkman (1983) according to which capacity investment can yield Cournot-consistent outputs in environments explicitly featuring price-setting competition. Cournot hypotheses alone are silent on the question of price formation. Once we explicitly account for price formation, the proposition that Cournot constitutes a conservative benchmark might prove to be less robust. Kreps and Scheinkman (KS) provide a theoretical foundation for linking price formation with investment in production capacity, and their results provide a rationale for appealing to Cournot. Yet other theoretical research suggests how the appeal to capacity constraints can yield behaviors that are richer than a Cournot-consistent hypothesis would predict. Edgeworth, for example, suggested that in the context of repeated interactions what Maskin and Tirole (1988) call "dynamic oligopoly" that capacity constraints can induce competitors to generate outputs and prices that cycle over time. (Edgeworth 1925, pp. 118-121) These cycles are the "Edgeworth Cycles" of Maskin and Tirole. The KS hypothesis has inspired much follow-on research, both theoretical and experimental, on whether or not Cournot-consistent outcomes do obtain in a wide range of environments. Theoreticians have pointed out that the KS hypothesis is sensitive to assumptions about the structure of residual demand (e.g. Davidson and Deneckere 1986). Specifically, KS depends on "efficient rationing" demand is assigned to the lowest-priced output. Introducing uncertainty in demand to the KS formulation with symmetric firms can also lead to multiple equilibria, no one of which may be symmetric (e.g., Reynolds and Wilson 2000). Yet other researchers have generalized the KS formulation by admitting the prospect that capacity may not be strictly constrained at the margin (e.g., Boccard and Wauthy 2000) or that firms may adjust capacity in the context of repeated oligopoly (Güth and Güth 2001). Either way, making capacity more elastic introduces more scope for symmetric Cournot outputs to obtain in equilibrium (Güth 1995). What do experiments have to say? In general, the results are consistent with the proposition that Cournot constitutes a conservative benchmark. In environments featuring efficient-rationing, Rassenti, Reynolds, Smith, and Szidarovsky (2000) observed average outputs that were Cournot-consistent or Cournot-superior. In an environment in which subjects explicitly chose both capacities and prices, Cournot-consistent or Cournot-superior capacities obtained on average (Muren 2000). In both of these environments, individual behaviors exhibited much heterogeneity. Anderhub, Güth, Kamecke and Normann (2003) also observe Cournot-consistent capacities in an environment featuring repeated oligopoly and staged investment. In their environment, subjects also chose both capacities and prices. Before giving the Cournot benchmark a complete pass, we should note at least one other set of complementary results. In related work, Rassenti and Wilson (2004) examine experimentally the role of the "dominant firm" and "competitive fringe firms" in a quantity-setting environment. Their point of reference is the Borenstein and Bushnell (1999) hypothesis. Borenstein and Bushnell pose a Cournot oligopoly of dominant firms and a fringe of price-taking firms. Thus, they maintain not so much that Cournot poses a conservative benchmark but that a dominant Cournot oligopoly with a price-taking fringe constitutes a conservative benchmark. Rassenti and Wilson simplify analysis by posing a single dominant firm rather than a group of dominant firms and find that the "dominant firm model" itself does not constitute a conservative benchmark in some respects.. Capacity is exogenous in their environment, but they do examine an environment in which all firms post prices. They also examine an environment in which subjects submit "offer curves" in a manner consistent with the supply function equilibrium approach of Klemperer and Meyer. They find that outputs often exceed the output implied by the dominant firm model, but they also find that prices often exceeded predicted prices. In the environment they examine, firms on the fringe do not behave as price-takers. A richer treatment would incorporate (1) endogenous capacity (2) explicit price setting and (3) active demand such as that encountered in a double auction. One only needs endogenous capacity and explicit price setting to craft tests of KS-type hypotheses, but no one experiment includes all three, including the environment we examine. Indeed, our environment only features endogenous capacity. That alone is not novel. What is novel is the extension to a richer set of production technologies. Our immediate purpose was not to craft tests of KS-type hypotheses but to generate results in a simpler environment that might yet scale up to a richer environment. As with other experiments, individual behaviors in our environment also exhibit much heterogeneity. We find that Cournot-consistent outputs obtain on average. 3. The Model We consider an environment in which agents can invest in two types of production capacity and in a risk-free security. The two types of capacity are analogous to "baseload" generation capacity and "marginal" (or "peaking") generation capacity in energy markets. Agents can use both types of capacity to produce output in a sequence of two markets. One market is characterized by "low" demand, and the other is characterized by "high" demand. We label these markets "Market L" and "Market H," respectively. Agents are endowed with investment resources. They must decide how much to invest in the risk-free security, in baseload capacity, and in marginal ("peaking") capacity before participating in markets L and H. They also must decide how much capacity of each type to dispatch in each of the two markets. The distinguishing feature of baseload capacity is that it is more expensive to install than marginal capacity, but it operates at lower marginal costs. We indicate the fixed costs of building capacity and the marginal costs of production as follows:
We assume FM < FB and CM > CB. Competition can be characterized as Cournot, and it is easy to characterize equilibria in which agents invest in some combination of baseload capacity, marginal capacity, and the risk-free security. We are principally interested in equilibria in which agents invest in both types of capacity and in the risk-free asset. We characterize competition by means of a static, constrained optimization problem. For any one agent, we let 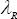 = investment in risk-free security = investment in risk-free security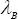 = investment in baseload capacity = investment in baseload capacity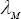 = investment in marginal capacity = investment in marginal capacity
r = the risk-free rate of return
The environment features N agents, each of whom faces residual demand functions PL and PH where  = demand in the low-demand state where = demand in the low-demand state where 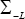 indicates the capacity dispatched by all other N - 1 agents. indicates the capacity dispatched by all other N - 1 agents.
The agent's choice variables are The corresponding maximization program is subject to 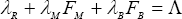
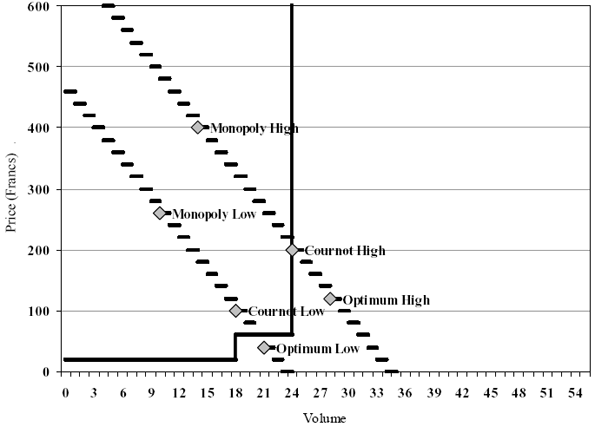
and complementary slackness conditions. It is possible to characterize a symmetric, pure-strategy equilibrium in which agents invest in both types of capacity and in the risk-free security ( The equilibrium corresponds to the following graph of supply and demand:
The following three lemmas yield the result. The first lemma indicates necessary supply-side conditions for the symmetric equilibrium. These supply-side conditions are not sufficient for yielding the proposed symmetric equilibrium and must be complemented with demand-side conditions. Even so, the supply-side conditions do restrict the best-replies that can obtain in any pure-strategy equilibrium, symmetric or asymmetric. The second lemma indicates these restrictions. The third lemma indicates demand-side conditions in an environment featuring linear demands that rule out equilibria in which agents' investments in baseload or marginal capacity are financially constrained. Lemma 1: A symmetric equilibrium in which each agent chooses ( 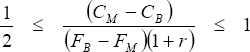 or, equivalently, 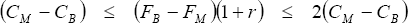 . .See Appendix 1. The result does not depend on the specific forms of the demand functions PL and PH. Lemma 1 assures that all of the shadow prices in the proposed symmetric equilibrium are non-negative. It indicates that neither marginal capacity nor baseload capacity can be too expensive relative to the other. Otherwise, agents might invest in only one type of capacity. More generally, one can show that a symmetric equilibrium in which agents only invest in marginal capacity exists only if Lemma 2: Given
See Appendix 2. Again, the result does not depend on the specific forms of the demand functions PL and PH. We now impose linear demand functions Lemma 3: Given the premise of lemma 2, the system of linear demands, and
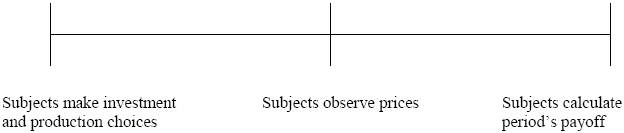
Note that item (ii) in lemma 3 constitutes a refinement of item (ii) in lemma 2. See Appendix 3. We can now pose conditions under which the proposed symmetric equilibrium is the only symmetric equilibrium. Each agent's maximand is weakly concave in that agent's seven choice variables, and the constraints are convex (linear), so we can appeal to a concave programming theorem according to which the first-order conditions for a maximum characterize a global maximum. The various supply-side and demand-side conditions rule out other candidate symmetric equilibria as well as equilibria in which investments in production capacity are financially constrained. Proposition 1: Assume One can choose See Appendix 4. Remark 1: We determine the joint-profit maximizing (monopoly) benchmark by evaluating all of the results in Lemma 3 for N = 1. Remark 2: We determine the social optimum benchmark by substituting the maximand with Most of the previous results obtain. (See Appendix 5.) Imposing the assumption of price-taking on the part of producers also yields the social optimum benchmark, although the prices that obtain in both the low demand state and the high demand state exceed the marginal costs of production. (See Figure 2.) 4. Experimental Design(5) The experiment features two treatments. In one treatment, subjects participated in a market featuring two production technologies and two demand conditions. The two technologies correspond to marginal and baseload capacity in the model, and the two demand conditions correspond to the two markets, L and H. The other treatment featured only one technology, but it also featured two demand conditions. The modeling presented here guided the design of the treatment with 2 technologies and 2 markets ("Treatment 2"), and we crafted a similar model, not presented here, to guide the design of the treatment with 1 technology and 2 markets ("Treatment 1"). We used the supply-side and demand-side conditions indicated in lemmas 2 and 3 to choose parameters for Treatment 2, and we used the supply-side and demand-side conditions indicated by the other model to choose parameters for Treatment 1. The French experimental subjects were endowed with investment resources enumerated in an experimental currency called "Yen." (Ironically enough, in the English-language version, subjects use an experimental currency called "Francs.") Subjects could use their Yen to buy "tickets." Subjects were informed that they could "redeem" any one ticket in one or both markets. Redeeming a ticket involved selling one unit of an experimental good called "units" in either or both of two markets. The markets were labeled "Market 1" and "Market 2." Market 1 corresponded to the low-demand state, and Market 2 corresponded to the high-demand state. Subjects could buy tickets from the experimenter at prices ("Purchase Prices") the experimenter publicly posted. The experimenter also posted "Redemption Fees" fees subjects would pay when and if subjects they chose to sell units. The Purchase Prices and Redemption Fees are analogous to fixed costs and marginal costs, respectively. The experiment did not feature active buyers on the demand side. Rather, the experimenter publicly posted a market demand schedule ("Redemption Schedule") for each of the two markets. Treatment 2 featured two types of tickets labeled Blue Tickets (bleue) and White Tickets (blanche) The Purchase Prices and Redemption Fees were structured so that Blue Tickets were analogous to units of baseload capacity and that White Tickets were analogous to marginal capacity. Treatment 1 only featured one type of tickets, although these tickets also featured a Purchase Price and a Redemption Fee. The experimenter posted the purchase prices, redemption fees and redemption schedules before subjects made their investment and production decisions. Subjects then made investment decisions and production decisions for both markets simultaneously. Investment decisions involved decisions to buy tickets, and production decisions amounted to choosing how many units to sell in each market. The experimenter aggregated subjects' choices and reported market outcomes. This sequence of posting prices, fees and schedules and aggregating decisions constituted a market period. We conducted several market periods in each of the six experimental sessions, and we did not vary the system parameters across sessions or across market periods. Figure 1 indicates the timing of decisions and payoffs in a market period, and Table 1 indicates the system parameters we used for the two treatments. Table 2 indicates investments, outputs and prices corresponding to three theoretical benchmarks. The benchmarks correspond to the equilibrium prediction (the "Cournot benchmark"), to the "Monopoly benchmark," and to the "Social Optimum benchmark." The entire demand system and the benchmarks for Treatment 2 are graphically represented in Figure 2. The supply function indicates the supply that obtains under the Cournot benchmark. The demand system is comprised of two step-functions, one indicating low demand and the other indicating high demand. 5. Results We conducted six experimental sessions between April 25 and October 3, 2005. Three sessions featured Treatment 1, and the other three sessions featured Treatment 2. All sessions featured 6 subjects. All subjects participated in a number of practice market periods. The practice environment featured only one technology and one market. All subjects were engineering students at the Institut Nationale Polytechnique de Grenoble. Table 3 indicates the number of market periods we dedicated to Treatments 1 or 2 with each set of subjects. Most of the results we report here are static in that we ignore dynamic considerations such as "learning." While it is reasonable to suggest that learning is an important phenomenon, it is interesting to suggest that treating realizations of output in each market period as independent events may nonetheless characterize the most important action. Indeed, the results are amenable to a simple, static narrative. Subjects behaviors exhibit much diversity, but, collectively, their behaviors are structured, stable, and susceptible to analysis with most orthodox and accessible game-theoretic concepts. Overall, they invest in capacity and dispatch capacity in ways consistent with the Nash-Cournot hypothesis. Even so, they do deviate from the Cournot benchmark in a systematic way. They tend to overinvest in marginal capacity and underinvest in baseload capacity. What this means is that consumers extract the Cournot-consistent surplus from the system but producers extract less than the Cournot-consistent surplus. They dissipate surplus by investing inefficiently; they could achieve Pareto improvements by investing less in marginal capacity and more in baseload capacity. The results indicate that the Cournot benchmark is a good indicator of outputs in both Treatments 1 and 2. This means that the Cournot benchmark also provides a good indicator of consumer surplus, since consumer surplus tracks output. Even so, this does not necessarily imply that the Cournot benchmark tracks producer surplus. Producer surplus also depends on the mix of subjects' investments. It turns out that the Cournot benchmark is a good indicator of subjects' investments in Treatment 1, but in Treatment 2 subjects consistently invest suboptimally, thus dissipating rents that they could otherwise have extracted for themselves. They consistently overinvest, relative to the Cournot benchmark, in marginal capacity and underinvest in baseload capacity. We should note that where the Cournot benchmark does perform well, behaviors do not converge on the Cournot benchmark but rather cycle around it. We will close this section by indicating a formative result about the manner in which behaviors cycle. The time series of producer surplus exhibit "sawtooth patterns," patterns that are suggestive of Edgeworth Cycles (Maskin and Tirole 1988). Even though producer surplus cycles, total system efficiency is much more stable. Subjects consistently generate total surplus that falls just short of the total surplus consistent with the Cournot benchmark. We break down these conclusions into seven discrete results. Result 1: Outputs cycle around the Cournot benchmarks. Figure 3 displays the cycling of both outputs and prices in Session 4 around the Cournot benchmarks for both Markets L and H. Price is plotted against the axis on the left, and output is plotted against the axis to the right. A single, horizontal line indicates the Cournot benchmark of (price, output) = (200, 24) for Market H, and a single dashed line indicates the Cournot benchmark of (100, 18) for Market L. The time series of both prices and outputs do not "converge" to the benchmarks, although they do line up with the benchmarks at different times during the session. What is striking, however, are not the time series but the averages of these quantities, especially the averages of the outputs. In Table 4 we present tests of the differences between the benchmark outputs and averages of outputs in the six experimental sessions. The third and fourth columns indicate both the averages of and bootstrapped standard errors of the outputs from each of the six experimental sessions.(6) The other columns to the right indicate the theoretical benchmarks and t-statistics corresponding to tests of the differences between the benchmarks and averages of the outputs. Both the Monopoly and Optimum benchmark outputs are statistically distinguishable from the realized average outputs. The Cournot benchmarks are not statistically distinguishable in all 7 of 12 instances and outperform the Monopoly benchmark in all 12 instances. The average output lies closer to the Optimum than to the Cournot benchmark in only one instance (Session 2, Market L). In the other 11 instances, the Cournot benchmark performs better. Result 2: Consumer surplus cycles around the Cournot benchmark. This result follows from the fact that output cycles around the Cournot benchmark. Even so, we present a complementary set of tests. Table 5 indicates t-tests for differences between average consumer surplus achieved in each session and the theoretical benchmarks. Again, we cannot statistically distinguish the Cournot benchmark from the actual surplus realized in each session. We can distinguish the Monopoly benchmark from the actual consumer surplus. The Social Optimum performs better than the Monopoly benchmark, but the Cournot benchmark dominates both benchmarks. Result 3: In Treatment 1, investment cycles around the Cournot benchmark. We present tests in Table 6. The investments in the first three sessions average about 24 units the number of units corresponding to the Cournot benchmark. In Sessions 1 and 2, these averages are not significantly different than the Cournot benchmark , but in session 3 investment is significantly lower than the Cournot benchmark. Average capacity investment is sharply distinguishable from the Monopoly and Social Optimum benchmarks in all three sessions. Result 4: In Treatment 2, subjects overinvest in marginal capacity and underinvest in baseload capacity, but the Cournot benchmark provided some indication of the total number of units invested. Again, see Table 6. None of the benchmarks characterize investment separately in baseload capacity or marginal capacity in that subjects invest much more heavily in marginal capacity and much less heavily in baseload capacity than any one benchmark would predict, but the total investment is not distinguishable from the Cournot benchmark of 24 unites in session 4. In each of the three sessions 4, 5, and 6, total investment exceeded the Cournot benchmark. We speculate that subjects overinvested in marginal capacity, because some of them perceived fixed costs as marginal. Marginal capacity may have featured a fixed cost of 50 francs, but the sum of the fixed and marginal costs was 110 francs. (See the system parameters in Table 1.) In contrast, baseload capacity may have featured a higher fixed cost of 100 francs, but the sum of the fixed and marginal costs was 120 francs. If one perceives the sum of fixed costs and marginal costs as marginal, then marginal capacity appears to dominate baseload capacity. Result 5: In Treatment 2, whether or not subjects have invested efficiently, they tend to dispatch capacity efficiently. Given subjects invest in some portfolio of baseload and marginal capacity, the prediction is that if they withhold any capacity, they withhold marginal capacity. Moreover, if they withhold capacity, they withhold capacity in the low demand state, Market L. Table 7 indicates the average number of units subjects withheld in both markets of each session. In the first three sessions, efficient dispatch entails withholding capacity in the Low Demand state and dispatching all capacity in the High Demand state. Sure enough, subjects withheld capacity in the low state and tended to dispatch all capacity in the high state. In Treatment 2 efficient investment entailed dispatching all baseload capacity in the law state and exhausting all baseload and marginal capacity in the high state. In Session 6 subjects did not, on average, exhaust all baseload capacity in the low state or all marginal capacity in the high state, but in all other instances subjects tended to exhaust baseload and marginal capacity when they should, and they withheld marginal capacity in the low state. Result 6: Producer surplus cycles between the Cournot and Social Optimum benchmarks, and it cycles in a manner suggestive of Edgeworth Cycles. In Figure 4 we represent the time series of producer surplus from Session 4. Producer surplus tends to decline to the Social Optimum benchmark over a number of period before rebounding in a single period to the Cournot benchmark. The pattern is suggestive of "sawtooth patterns" or Edgeworth Cycles others have observed in markets such as those for retail gasoline. (See, for example, Noel 2004.) In retail gasoline markets, prices tend to fall slowly before rebounding to a higher level and slowly falling again. It would be hard to suggest that the price data generated in this experiment exhibit Edgeworth Cycles, but producer surplus provides a way of aggregating the performance of more than one market in a single metric. It is this single metric, producer surplus, that exhibits sawtooth patterns. The time series of producer surplus exhibited in the other 5 sessions do not necessarily exhibit such a clear pattern, although those data are also suggestive. The results, suggest that cycling patterns may yet be amenable to further experimental analysis. Result 7: Total system stability and efficiency. The time series of total system efficiency is more stable than the series or either producer surplus or consumer surplus a reflection of the fact that consumer surplus and producer surplus are negatively correlated. (See Figure 5.) The standard errors of total surplus are much smaller than the standard errors that correspond to consumer surplus. Compare, for example, the standard errors reported in Table 8 to those reported in Table 5. In both treatments, system efficiency falls short of the Cournot benchmark and is sharply distinguishable from the Monopoly benchmark. The Social Optimum benchmark indicates the surplus available in the system. In each of the six sessions, system efficiency exceeds 94%. In Table 8 we indicate the total surplus extracted, on average, in each session. System efficiency falls short of the Cournot benchmark and is statistically distinguishable from all three benchmarks. 6. Conclusion The experimental framework constitutes a first step in crafting a richer platform for exploring how investment interacts with market power, market structure, and market institutions. The existing framework features the simplest environment we could construct for exploring an investment problem that involves more than one production technology. We used it to generate accessible, discrete results. The results are interesting in themselves, but, more importantly, they provide a baseline against which to compare results in richer environments. The framework can be adapted for examining investment behaviors in richer environments. We can, for example, adapt the framework to explore how investment varies across different market structures and different institutional landscapes. Specifically, we can add more and differently-endowed players, and we can use different market institutions. The framework can also be adapted to include investment in innovation. Parties might, for example, not merely invest in production capacities but may devote some resources to developing and commercializing new production technologies. Making the environment richer leads to questions about how far baseline results scale up. As John Ledyard (1993) suggests by metaphor, results achieved with scaled-down models in the engineer's wind tunnel or towing tank may not correspond to those achieved with full-size prototypes in the open skies or open waters. Experimental planes crash and vessels capsize. But there are two motivations for starting small and simple. First, more ambitious experiments and research might frustrate efforts to identify and isolate baseline effects that do scale up. Researchers might become distracted by noise produced in richer environments and miss the fundamental action. Second, baseline results can inform analysis of results achieved in richer environments. With baseline results in hand, one can always pose the hypothesis that baseline results have scaled up and can work out implications from there. The hypothesis is interesting if it helps to reveal new results. It is also interesting if posing it leads to implausible conclusions, in which case one might accept the alternative hypothesis that results do not scale up. The existing framework has allowed us to yield a number of simple, baseline results. Parties dispatch capacity efficiently, but they do not invest efficiently. They overinvest in "marginal" capacity and underinvest in "baseload" capacity. Inefficient investment depresses total system efficiency. Even so, consumers extract the surplus that is available to them in the game-theoretic benchmark. The game-theoretic benchmark itself is interesting, because it helps distinguish outcomes from alternative benchmarks. These benchmarks include a monopoly benchmark and a socially optimal benchmark. The results suggest some immediate follow-on research. First, sequencing output decisions in the two markets might allow subjects to figure out how to invest more efficiently. Specifically, we would allow them to experience outcomes in one of the two markets before making output decisions for the second market. Would they be better able to internalize the nature of fixed and marginal costs in the system? Second, the existing framework is amenable to exploring dynamic oligopoly. Sawtooth patterns manifest themselves in certain measures of system performance. Generating longer times series can allow us to investigate the robustness of these patterns and to suggest explanations for them that might inform theories of oligopolistic competition. References Anderhub, Vital, Werner Güth, Ulrich Kamecke, and Hans-Theo Normann. "Capacity Choices and Price Competition in Experimental Markets." Experimental Economics 6 (2003): 27-51. Banks, Jeffrey S., John O.Ledyard, and David P. Porter. "Allocating Uncertain and Unresponsive Resources: An Experimental Approach." RAND Journal of Economics 20 (1989): 1-25. Blumstein, Carl, Lee S. Friedman, and Richard J. Green. "The History of Electricity Restructuing in California." Journal of Industry, Competition and Trade 2 (2002): 9-38. Boccard, Nicolas and Xavier Wauthy. "Bertrand Competition and Cournot Outcomes: Further Results." Economics Letters 68 (2000): 279-285. Borenstein, Severin, James B. Bushnell, and Frank A. Wolak. "Measuring Market Inefficiency in California's Wholesale Electricity Market." American Economic Review 92 (2002): 1376-1405. Borenstein, Severin, and James B. Bushnell. "An Empirical Analysis of the Potential for Market Power in California's Electricity Industry." Journal of Industrial Economics 47 (1999): 285-323. Davidson, Carl, and Raymond Deneckere. "Long-run Competition in Capacity, Short-run Competition in Price, and the Cournot Model," RAND Journal of Economics 17 (1986): 404-415. Edgeworth, Francis Y. Papers Relating to Political Economy, Volume 1. Macmillan (1925) Green, Richard J. and David M. Newbery. "Competition in the British Electricity Spot Market." Journal of Political Economy 100 (1992): 929-953. Güth, Sandra and Werner Güth. "Preemption in Capacity and Price Determination: A Study of Endogenous Timing of Decision for Homogeneous Markets." Metroeconomica 52 (2001): 329-355. Güth, Werner. "A Simple Justification of Quantity Competition and the Cournot-Oligopoly Solution." ifo Studien 17 (1995): 245-257. Joskow, Paul and Edward Kahn. "A Quantitative Analysis of Pricing Behavior in California's Wholesale Electricity Market During Summer 2000." The Energy Journal 23 (2002): 1-35. Klemperer, Paul D. and Margaret A. Meyer. "Supply Function Equilibria in Oligopoly under Uncertainty." Econometrica 57 (1989): 1243-1277. Kreps, David, and Jose Scheinkman. "Quantity Precommitment and Bertrand Competition Yield Cournot Outcomes." Bell Journal of Economics 14 (1983): 326-337. Ledyard, John O. "The Design of Coordination Mechanisms and Organizational Computing." Journal of Organizational Computing 3 (1993): 121-134. Maskin, Eric and Jean Tirole. "A Theory of Dynamic Oligopoly II: Price Competition, Kinked Demand Curves and Edgeworth Cycles." Econometrica 56 (1988): 571-599. Muren, Astri. "Quantity Precommitment in an Experimental Oligopoly." Journal of Economic Behavior and Organization 41 (200): 147-157. Noel, Michael. "Edgeworth Price Cycles: Evidence from the Toronto Retail Gasoline Market." unpublished manuscript posted at http://repositories.cdlib.org/ucsdecon/2004-03. (2004). Plott, Charles R. "Market Architectures, Institutional Landscapes and Testbed Experiments." Economic Theory 4 (1994): 3-10. Rassenti, Stephen J., Stanley S. Reynolds, Vernon L. Smith and Ferenc Szidarovszky. "Adaptation and Convergence of Behavior in Repeated Experimental Cournot Games." Journal of Economic Behavior and Organization 41 (2000): 117-146. Rassenti Stephen J., and Bart J. Wilson. " How Applicable is the Dominant Firm Model of Price Leadership?" Experimental Economics 7 (2004): 271-288. Reynolds, Stanley S. and Bart J. Wilson. "Bertrand-Edgeworth Competition, Demand Uncertainty, and Asymmetric Outcomes." Journal of Economic Theory 92 (2000): 122-141. Roques, Fabien A., David M. Newbery and William J. Nuttall. "Investment Incentives and Electricity Market Design: The British Experience." Review of Network Economics 4 (2005): 93-128. Wolak, Frank A. and Robert H. Patrick. "The Impact of Market Rules and Market Structure on the Price Determination Process in the England and Wales Electricity Market." NBER Working Paper 8248 (2001) Wolfram, Catherine. "Measuring Duopoly Power in the British Electricity Spot Market." American Economic Review 89 (1999): 805-826. Timing of Subjects’ Decisions in One Market Period Treatment 2: The Experimental System Total Surplus and Consumer Surplus
* The quantities are denominated in the experimental currency.
Number of Market Periods in each Experimental Session
The notations ***, **, and * indicate statistical significance at the 1%, 5%, and 10% levels, respectively. Tests based on bootstrapped confidence intervals or bootstrapped standard errors yield the same results.
The notations ***, **, and * indicate statistical significance at the 1%, 5%, and 10% levels, respectively. Tests based on bootstrapped confidence intervals or bootstrapped standard errors yield the same results. Proportions of Capacity Withheld
Total System Efficiency
The corresponding Lagrangian is The Kuhn-Tucker conditions are:
and 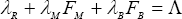
complementary slackness conditions. The equilibrium conditions and the complementary slackness conditions indicate conditions on the multipliers: 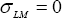 implies implies  and and 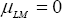 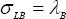 implies implies 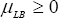 and and 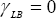 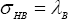 implies implies 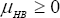 and and 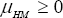 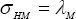 implies implies 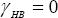 and and 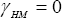 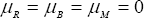 These conditions and (1) imply
Substituting (8) into (11) and rearranging yields
Similarly, substituting (8) into (10) yields
Inequalities (13) and (15) achieve the result. Remark: All of the other multipliers are non-negative. We only need to solve for Under the system of linear demands, the first seven of the agent's first-order conditions become:
Equation (1) implies
Subtracting (4) from (5) yields
Taking the difference of these last two expressions and rearranging yields which implies
This last expression (A) implies that given an agent dispatches baseload capacity in the low state, the agent also exhausts all of that baseload capacity in the high state. Now, by similar reasoning, subtracting the difference between (6) from (7) from the difference between (3) and (2) yields which implies
This last expression implies that given an agent dispatches baseload capacity in the high state, the agent also exhausts all of that baseload capacity in the low state. Thus, feasibility constraints and equations (A) and (B) together imply result (i): To establish result (ii), we simply examine equation (3). Equation (3) indicates that
This last inequality and complementary slackness conditions imply
Thus, either We again take the difference between equations (4) and (5) and add to it the difference between (6) and (7). We then subtract from this sum the difference between (2) and (3) to yield This last expression implies
Equation (D) indicates that given agent invests some resources in the risk-free asset (in which case We now state without proof that one can choose
This last expression and equation (C) achieve the result. We also impose The first seven first-order conditions become
Equation (1) implies Equation (2) yields Equations (4) and (5) yield Both Equation (4) alone yields We achieve the last two inequalities by imposing Equation (6) implies 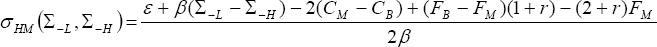 . .We achieve the last two inequalities by imposing The term The maximand is (weakly) concave in the choice variables, and the constraints are linear in the choice variables. We conclude that the first-order conditions not only satisfy second-order conditions for a local maximum but also characterize a global maximum. We now outline how to rule out the five other candidate symmetric equilibria. One can break candidate equilibria (i) and (ii) by indicating that a single agent can profitably (and optimally) deviate by setting In candidate equilibrium (iii), one can show that
As before, we get the inequalities
The Schedule of Redemption Values may or may not change from one Market Period to the next.
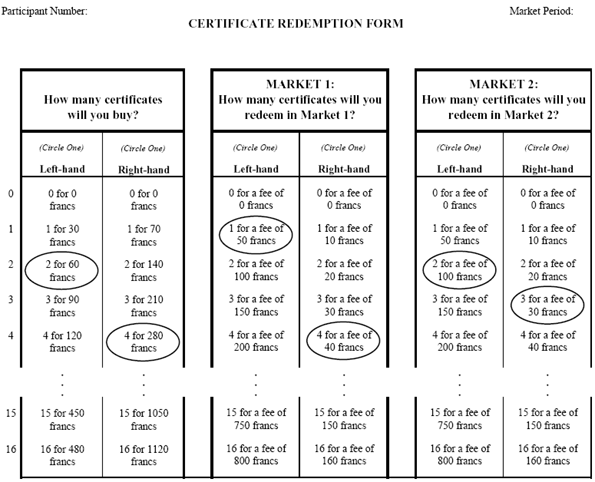
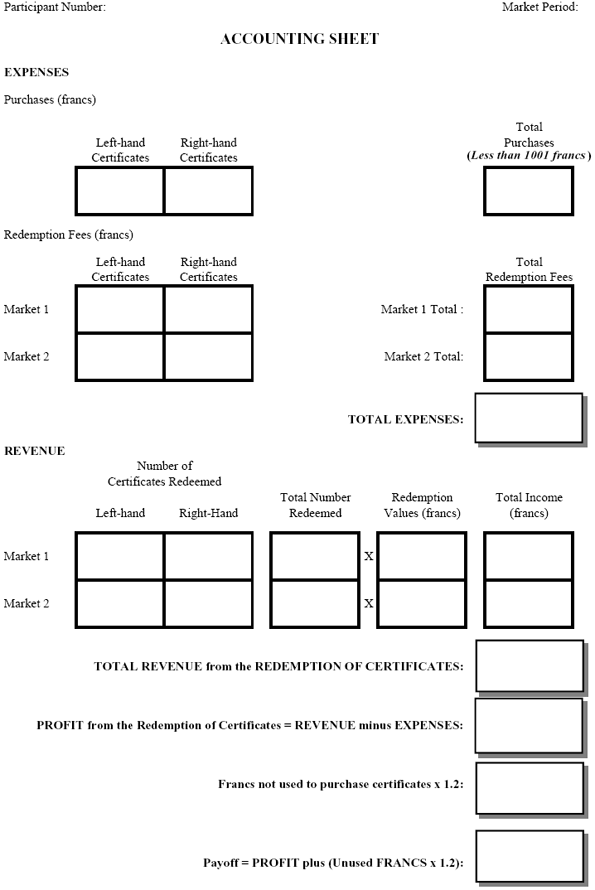
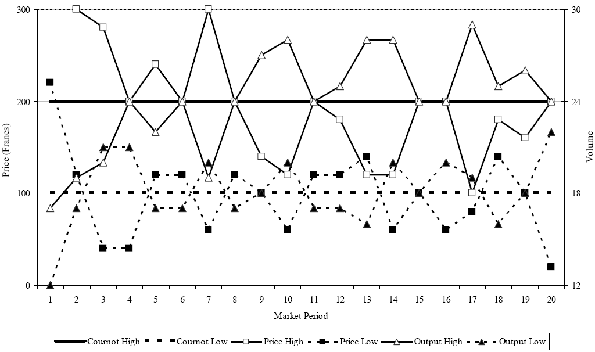
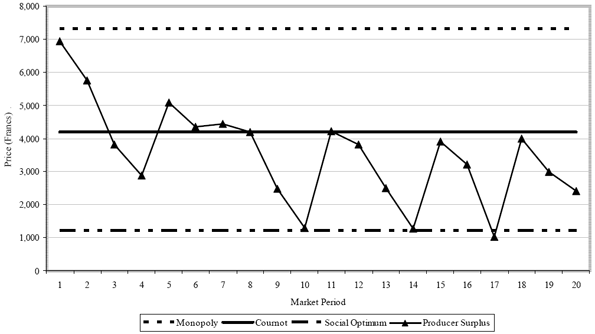
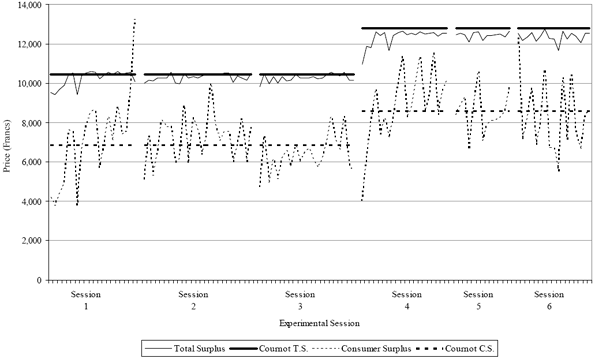
In the example indicated below, a participant may choose to buy two Left-hand Certificates for a total price of 60 francs by circling the option "2 for 60 francs" in the column labeled "Left-hand" under the caption "How many certificates will you buy?" The participant may buy four Right-hand Certificates by circling the option "4 for 280 francs" in the column labeled "Right-hand" under the caption "How many certificates will you buy?" Similarly, under the captions "MARKET 1" and "MARKET 2," you will indicate how many Left-hand and Right-hand Certificates you would like to redeem in the two markets. Given a participant chooses to buy two Left-hand Certificates, he or she can redeem as many as two Left-hand Certificates, but not more than two Left-hand Certificates in Market 1 and in Market 2. In the example indicated above, the participant has chosen to redeem one Left-hand Certificate in Market 1 for a fee of 50 francs and two Left-hand Certificates in Market 2 for a fee of 100 francs. Altogether the participant used two Left-hand Certificates to sell three units. Similarly, the decision to buy four Right-hand Certificates enables the participant to redeem as many as four Right-hand Certificates in Market 1 and in Market 2. In the example indicated above, the participant has chosen to redeem four Right-hand Certificates in Market 1 for a fee of 40 francs and to redeem three Right-hand Certificates in Market 2 for a fee of 30 francs. Altogether, the participant used the four Right-hand Certificates to sell seven units. Each participant will make his or her selections secretly. The experimenter will collect all participants' forms, determine the numbers of Left-hand and Right-hand Certificates redeemed in Markets 1 and 2, and find the corresponding prices on the Schedules of Redemption Values. The experimenter will publicly report the prices at which the certificates will be redeemed and will report the total number of Left-hand Certificates and Right-hand Certificates redeemed in each market. The experimenter will return each participant's Certificate Redemption Form, and participants will calculate their earnings for the Market Period on an Accounting Sheet. (See below.) On this sheet the participant will record the francs they used to buy certificates and the francs they used to pay redemption fees. The sum of purchases and redemption fees constitutes "Total Expenses" for the Market Period. Participants will also use this sheet to record the revenues they gained from selling units on Markets 1 and 2. You calculate your revenue from a market by multiplying the sum of all Left-hand and Right-Certificates you redeemed in that market by the Redemption Value that obtained in that market. "Total Revenue" is comprised of the revenue gained from both markets. Finally, you will calculate the payoff you earned on francs you did not use to buy certificates. Your total income, then, will be comprised of your Total Revenue from the sale of units, the payoff you earned on francs not used to buy certificates, and the francs you did not use. The payoff you gain from the entire Market Period is the difference between your total income and your total expenses. Participants will use one Certificate Redemption Form and one Accounting Sheet for each Market Period. At the end of the experiment, you will add up the payoffs from each of the Market Periods. The resulting sum will constitute your total payoff for participating in the experiment. The experimenter will convert your francs into dollars at a rate of 2,000 francs to the dollar. You will not be able to apply payoffs earned in previous Market Periods to your participation in succeeding Market Periods. In each Market Period your participation will depend only on the 1,000 francs the experimenter gives you. FOOTNOTES We thank Dominique Finon, participants in the 2004 meetings of the International Society for New Institutional Economics, and seminar participants at the Federal Reserve Bank of Dallas, the Interdisciplinary Center for Economic Science at George Mason University, Purdue University, and West Virginia University for helpful comments and suggestions relating to an earlier incarnation of this paper. The usual disclaimers apply with one exception: The views expressed in this paper do not reflect those of the United States Department of Justice. 1. Contact Author. United States Department of Justice: dean.williamson@usdoj.gov. The views expressed in this paper do not reflect those of the United States Department of Justice. 2. Université de Grenoble: celine.jullien@ensgi.inpg.fr 3. International Foundation for Research in Experimental Economics (IFREE) and Department of Economics, Northwestern University: lkiesling@northwestern.edu, who gratefully acknowledges funding from the Searle Fund for Policy Research at Northwestern University and IFREE for this research. 4. Université Panthéon-Sorbonne: carine.staropoli@univ-paris1.fr 5. We include the experiment instructions in Appendix 6. We conducted the experimental sessions in Grenoble with a French translation of the instructions. 6. All standard errors and confidence intervals (not reported) we use in all of are exhibits were each generated from 10,000 bootstrap samples. As few as 100 bootstrap samples would have done the trick. |
||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||

 U.S. Department
of Justice
U.S. Department
of Justice
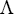 = agent's endowment of investment resources.
= agent's endowment of investment resources. = volume of baseload capacity dispatched in period L
= volume of baseload capacity dispatched in period L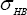 = volume of baseload capacity dispatched in period H
= volume of baseload capacity dispatched in period H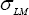 = volume of marginal capacity dispatched in period L
= volume of marginal capacity dispatched in period L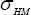 = volume of marginal capacity dispatched in period H
= volume of marginal capacity dispatched in period H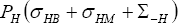 = demand in the low-demand state where
= demand in the low-demand state where  indicates the capacity dispatched by all other N - 1 agents.
indicates the capacity dispatched by all other N - 1 agents. ,
, 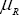 ,
, 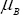 ,
, 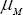 ,
, 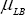 ,
, 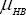 ,
,  ,
, 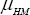 ,
, 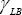 ,
, 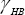 ,
, 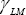 and
and 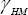 .
.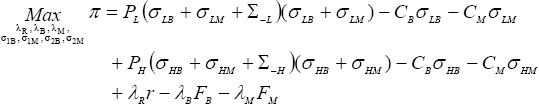
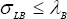
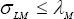
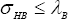
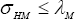
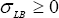

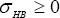
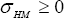

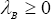
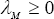
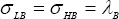 ), and they reserve marginal capacity in the low-demand state (
), and they reserve marginal capacity in the low-demand state (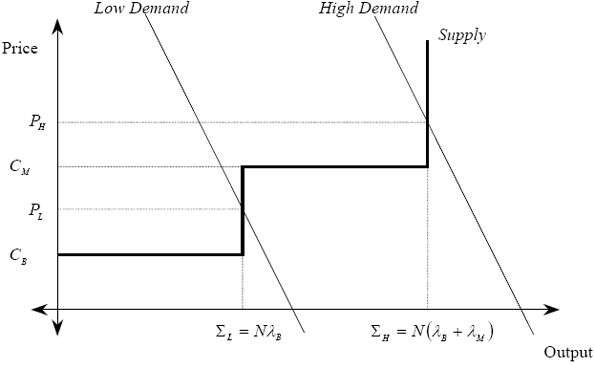
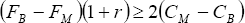 , and a symmetric equilibrium in which agents only invest in baseload capacity exists only if
, and a symmetric equilibrium in which agents only invest in baseload capacity exists only if 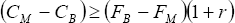 . Now we state results that do not depend on either symmetry or strategic interdependence.
. Now we state results that do not depend on either symmetry or strategic interdependence. , any one agent's strategy (
, any one agent's strategy (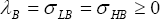 That is, given an agent invests in baseload capacity, the agent exhausts all of that capacity in both the low demand state and the high demand state. Agents do not let costly capacity go unused, and they use all of that capacity in both states.
That is, given an agent invests in baseload capacity, the agent exhausts all of that capacity in both the low demand state and the high demand state. Agents do not let costly capacity go unused, and they use all of that capacity in both states.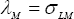 or
or  . That is, given an agent invests in marginal capacity, that agent exhausts all of that capacity in at least one of the two demand states. Agents do not let costly (marginal) capacity go unused.
. That is, given an agent invests in marginal capacity, that agent exhausts all of that capacity in at least one of the two demand states. Agents do not let costly (marginal) capacity go unused.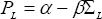 and
and 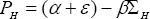 . The next lemma simply indicates that, given sufficiently large investment resources ?, it makes sense for the agent to invest some resources in the risk-free asset. That is, it indicates a condition under which investments in baseload and marginal capacity are not financially constrained.
. The next lemma simply indicates that, given sufficiently large investment resources ?, it makes sense for the agent to invest some resources in the risk-free asset. That is, it indicates a condition under which investments in baseload and marginal capacity are not financially constrained.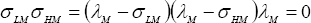 . That is,
. That is, 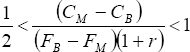 ,
, 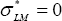 ,
,
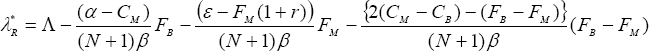
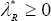 in which case the vector (
in which case the vector ( ,
,  ,
, 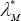 ,
, 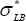 ,
, 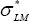 ,
, 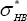 ,
, 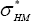 ) corresponds to a global maximum of each agent's program. Moreover, the profile of symmetric strategies constitutes the only symmetric equilibrium of the game.
) corresponds to a global maximum of each agent's program. Moreover, the profile of symmetric strategies constitutes the only symmetric equilibrium of the game.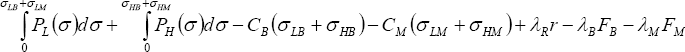 .
. 
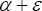

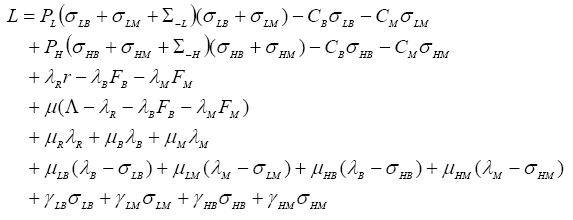
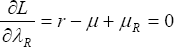
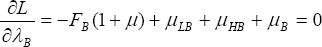
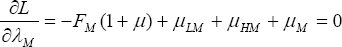

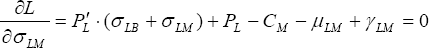
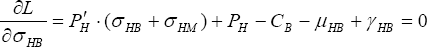
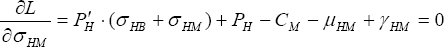
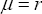 . Subtracting (2) from (3) and rearranging yields
. Subtracting (2) from (3) and rearranging yields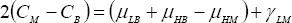
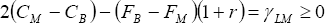
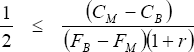
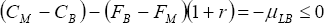
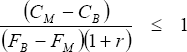
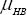 and
and 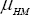 . Equations (2) and (12) yield
. Equations (2) and (12) yield 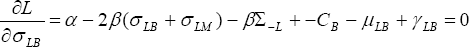
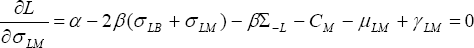

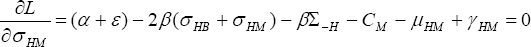
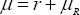 . Now, subtracting equation (3) from (2) and substituting
. Now, subtracting equation (3) from (2) and substituting 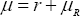 yields
yields 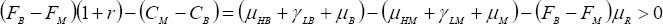
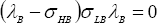
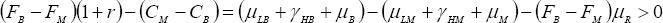
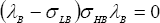 .
.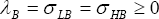 .
.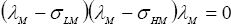 .
.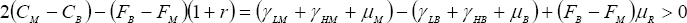
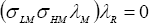
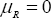 and the last inequality becomes
and the last inequality becomes 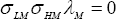 or, simply,
or, simply, 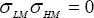
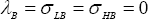
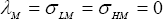
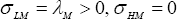
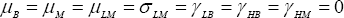 and must solve for
and must solve for 

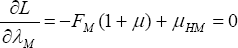
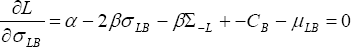
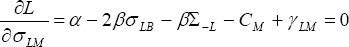
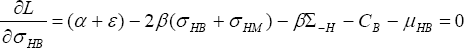
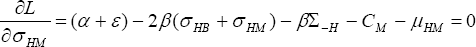
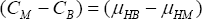 , and (3) implies
, and (3) implies 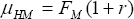 . These last two expressions yield
. These last two expressions yield 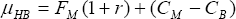 .
. 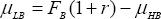 . Substitution into the previous expression yields
. Substitution into the previous expression yields 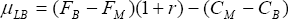 .
. 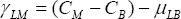 . Again, substitution into the previous expression yields
. Again, substitution into the previous expression yields 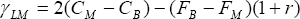 .
. 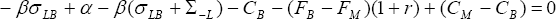 . Rearranging yields one of an agent's reaction functions:
. Rearranging yields one of an agent's reaction functions: 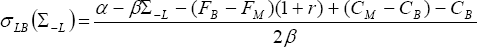 .
.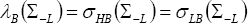 .
. 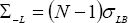 ,
, 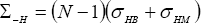 , and the equilibrium level of baseload capacity dispatched by each agent in the low state is
, and the equilibrium level of baseload capacity dispatched by each agent in the low state is 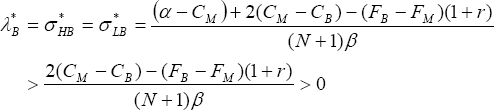 .
. 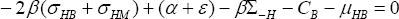 . Substituting
. Substituting 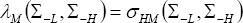 . So, in equilibrium
. So, in equilibrium 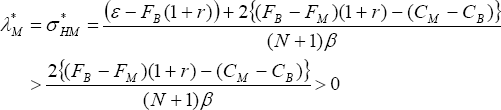 .
. 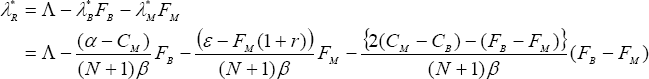
 but is increasing in
but is increasing in ![\lambda _M = \sigma _{HM} = \frac{{\left( {\alpha - C_M } \right) + \left[ {\varepsilon - F_M \left( {1 + r} \right)} \right]}}{{2\beta }}](/atr/public/eag/221877/170.gif) .
.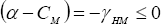 , which contradicts the premise that
, which contradicts the premise that 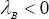 , another contradiction. Finally, solving for
, another contradiction. Finally, solving for 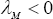 .
.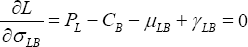
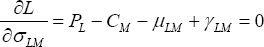
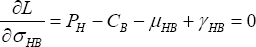
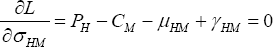
![\lambda _B = \sigma _{LB} = \sigma _{HB} = \frac{{\alpha - C_B + \left[ {(C_M - C_B ) - (F_B - F_M )(1 + r)} \right]}}{\beta }](/atr/public/eag/221877/179.gif)
![\lambda _M = \sigma _{HM} = \frac{{\varepsilon - \left[ {2(C_M - C_B ) - (F_B - F_M )(1 + r)} \right] - F_M (1 + r)}}{\beta }](/atr/public/eag/221877/180.gif)